Here is everything you need to know about simultaneous equations for GCSE maths (Edexcel, AQA and OCR). To be able to solve an equation like this, another equation needs to be used alongside it. That way it is possible to find the only pair of values that solve both equations at the same time. Linear simultaneous equations refer to simultaneous equations where the degree of the variables is one. Now, locate at least a couple of points (x, y) satisfying the equations to plot the straight-line graph. You can use an online tool such as graphsketch to draw the graphs and check your solution.

Graphing Method for Solving Simultaneous Equations

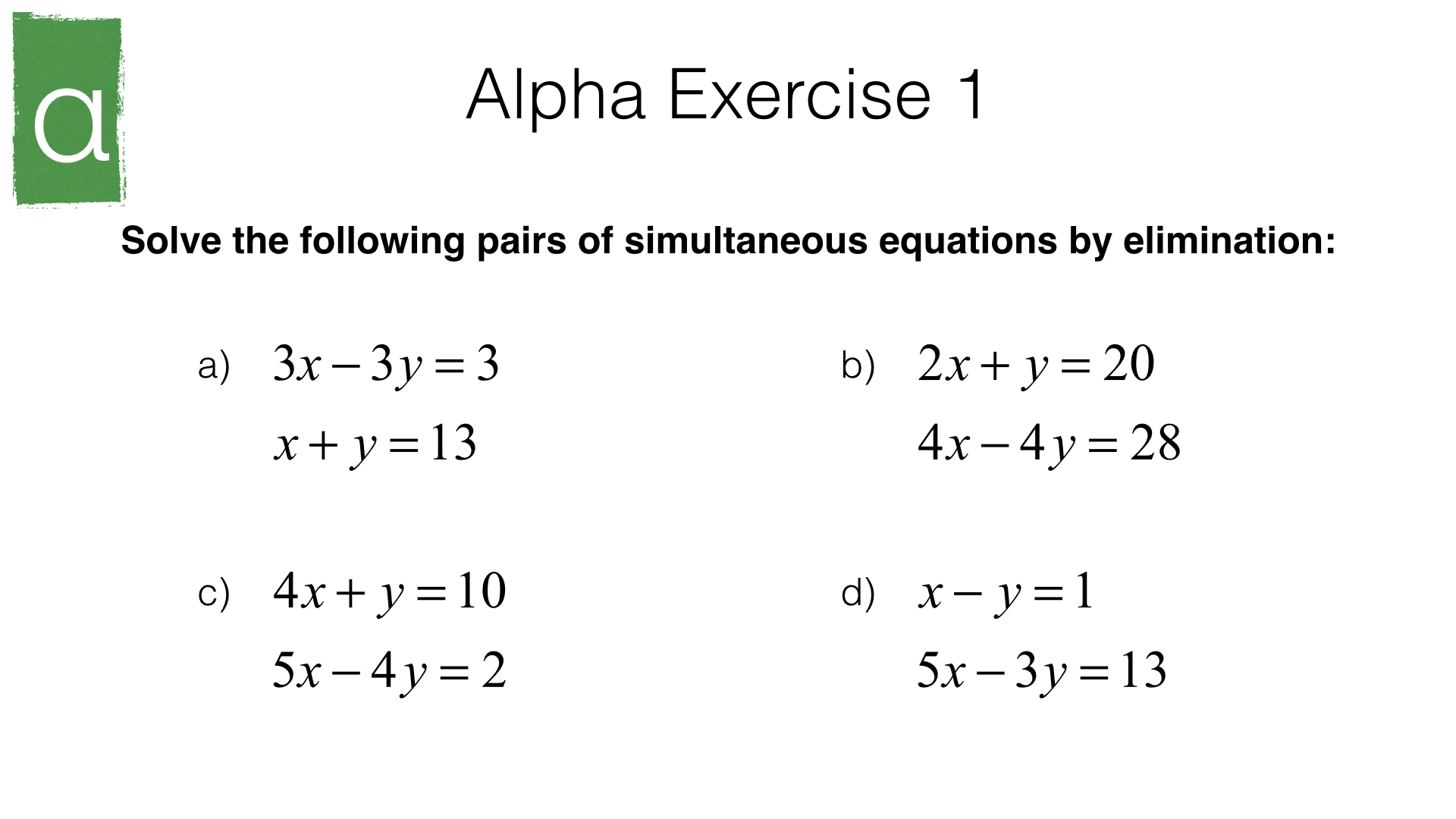
Sometimes equations need to be altered, by multiplying throughout, before being able to eliminate one of the variables (letters). In this case, a good strategy is to multiply the second equation by 3 . We can then subtract the second equation from the first to leave an equation with a single variable. Once this value is determined, we can substitute it into either equation to find the value of the other variable.

• That is if the simultaneous linear equations include only two variables, the cross-multiplication method can be used to find their solution.
• This fact can be used to help solve the two simultaneous equations at the same time and find the values of \(x\) and \(y\).
• Linear simultaneous equations are called those equations in which power of each unknown variable is one.
• You need to plot two straight lines using the equations, then see where they cross.

Substitute \(x\) back into either original equation and solve for \(y\)

There are well known three methods we use to solve simultaneous equations, as are listed below. Linear simultaneous equations are called those equations in which power of each unknown variable is one. We can consider each equation as a function which, when displayed graphically, may child tax credit schedule 8812 intersect at a specific point. This point of intersection gives the solution to the simultaneous equations. Apart from those methods, we can also the system of linear equations using Cramer’s rule. The following video shows how to solve simultaneous equations using substitution.

FAQs on Simultaneous Equations

In mathematics it is very important to find out the collect solution for carrying good grades. The cross-multiplication method is applied when we need to solve a pair of linear equations in two variables. That is if the simultaneous linear equations include only two variables, the cross-multiplication method can be used to find their solution.

4 Solving simultaneous equations (EMA

A pair of equations like this are called simultaneous equations – because you are trying to solve them both with the same values for \(x\) and \(y\). Quadratic simultaneous equations are solved by the substitution method. The number of variables in simultaneous equations must match the number of equations for it to be solved. You need to plot two straight lines using the equations, then see where they cross. The values of \(x\) and \(y\) at this point are the solutions of the simultaneous equations.

Evaluation Method for Solving Simultaneous Equations

The unknowns of \(x\) and \(y\) have the same value in both equations. This fact can be used to help solve the two simultaneous equations at the same time and find the values of \(x\) and \(y\). First of all, we draw the graph of both equation one by one and then trace out the intersection of lines, which will be the our required solution. Since it is a quadratic equation in term of ‘y’, we can solve it by factorization. In this step ‘x’ eliminates, we get the equation in term of ‘y’ only. You need to draw the graphs of the two equations and see where they cross.

We will get the value of a and b to find the solution for the same. Go through the following problems which use substitution and elimination methods to solve the simultaneous equations. To solve simultaneous equations, we need the same number of equations as the number of unknown variables involved. We shall discuss each of these methods in detail in the upcoming sections with examples to understand their applications properly. This is another approach to solving simultaneous linear equations in two variables.

Jon bought one packets of chips so, amount for chips will be ‘x’ and he bought seven packets of biscuits, amount for biscuits will be ‘7y’. Since values of x and y satisfy both equations, so our solution is correct. \(x\) and \(y\) values can be found which will solve both of the original equations at the same time or simultaneously. An equation with two unknown values will have infinitely many solutions. Subtracting the second equation from the first equation leads to a single variable equation. Use this equation to determine the value of y , then substitute this value into either equation to determine the value of x .

In this article, we will explore the concept of simultaneous equations and learn how to solve them using different methods of solving. We shall discuss the simultaneous equations rules and also solve a few examples based on the concept for a better understanding. From the graph, it is observed that the point of intersection of two straight lines is (2, 2), which is the solution for the given simultaneous linear equation. After finding out the value of one unknown variable we put this in any one equation and find out the other equations.